In my paper with É. Fouvry and Ph. Michel where we find upper bounds for the number of certain sheaves on the affine line over a finite field with bounded ramification, the combinatorial part of the argument involves spherical codes and the method of Kabatjanski and Levenshtein, and turns out to depend on the rather recondite question of knowing a lower bound on the size of the largest zero $latex x_n$ of the $latex n$-th Hermite polynomial $latex H_n$, which is defined for integers $latex n\geq 1$ by
$latex H_n(x)=(-1)^n e^{x^2} \frac{d^n}{dx^n}e^{x^2}.$

This is a classical orthogonal polynomial (which implies in particular that all zeros of $latex H_n$ are real and simple). The standard reference for such questions seems to still be Szegö’s book, in which one can read the following rather remarkable asymptotic formula:
$latex x_n=\sqrt{2n}-\frac{i_1}{\sqrt[3]{6}}\frac{1}{(2n)^{1/6}}+o(n^{-1/6})$
where $latex i_1=3.3721\ldots>0$ is the first (real) zero of the function
$latex \mathrm{A}(x)=\frac{\pi}{3}\sqrt{\frac{x}{3}}\Bigl\{J_{1/3}\Bigl(2\Bigl(\frac{x}{3}\Bigr)^{3/2}\Bigr)+J_{-1/3}\Bigl(2\Bigl(\frac{x}{3}\Bigr)^{3/2}\Bigr)\Bigr\}$
which is a close cousin of the Airy function (see formula (6.32.8) in Szegö’s book, noting that he observes the Peano paragraphing rule, according to which section 6.32 comes before 6.4).

(Incidentally, if — like me — you tend to trust any random PDF you download to check a formula like that, you might end up with a version containing a typo: the cube root of $latex 6$ is, in some printings, replaced by a square root…)

Szegö references work of a number of people (Zernike, Hahn. Korous, Bottema, Van Veen and Spencer), and sketches a proof based on ideas of Sturm on comparison of solutions of two differential equations.

As it happens, it is better for our purposes to have explicit inequalities, and there is an elementary proof of the estimate
$latex x_n\geq\sqrt{\frac{n-1}{2}},$
which is only asymptotically weaker by a factor $latex 2$ from the previous formula. This is also explained by Szegö, and since the argument is rather cute and short, I will give a sketch of it.

Besides the fact that the zeros of $latex H_n$ are real and simple, we will use the easy facts that $latex \deg(H_n)=n$, and that $latex H_n$ is an even function for $latex n$ even, and an odd function for $latex n$ odd, and most importantly (since all other properties are rather generic!) that they satisfy the differential equation
$latex y”-2xy’+2ny=0.$

The crucial lemma is the following result of Laguerre:

Let $latex P\in \mathbf{C}[X]$ be a polynomial of degree $latex n\geq 1$. Let $latex z_0$ be a simple zero of $latex P$, and let
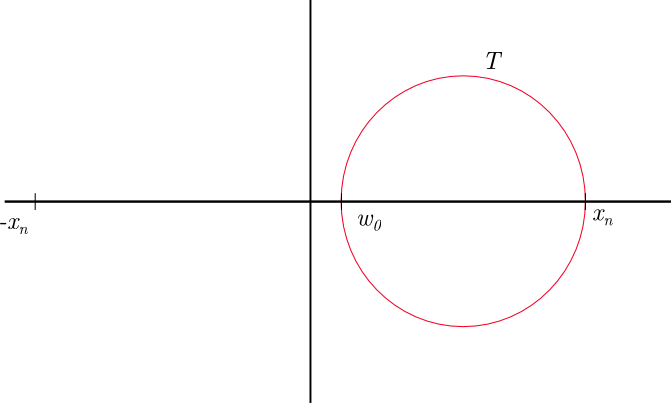
$latex w_0=z_0-2(n-1)\frac{P'(z_0)}{P”(z_0)}.$
Then if $latex T\subset \mathbf{C}$ is any line or circle passing through $latex z_0$ and $latex w_0$, either all zeros of $latex P$ are in $latex T$, or both components of $latex \mathbf{C}-T$ contain at least one zero of $latex P$.

Before explaining the proof of this, let’s see how it gives the desired lower bound on the largest zero $latex x_n$ of $latex H_n$. We apply Laguerre’s result with $latex P=H_n$ and $latex z_0=x_n$. Using the differential equation, we obtain
$latex w_0=x_n-\frac{n-1}{x_n}.$
Now consider the circle $latex T$ such that the segment $latex [w_0,z_0]$ is a diameter of $latex T$.

Now note that $latex -x_n$ is the smallest zero of $latex H_n$ (as we observed above, $latex H_n$ is either odd or even). We can not have $latex w_0<-x_n$: if that were the case, the unbounded component of the complement of the circle $latex T$ would not contain any zero, and neither would $latex T$ contain all zeros (since $latex -x_n\notin T$), contradicting the conclusion of Laguerre's Lemma. Hence we get $latex -x_n\leq w_0=x_n-\frac{n-1}{x_n},$
and this implies
$latex x_n\geq \sqrt{\frac{n-1}{2}},$
as claimed. (Note that if $latex n\geq 3$, one deduces easily that the inequality is strict, but there is equality for $latex n=2$.)

Now for the proof of the Lemma. One defines a polynomial $latex Q$ by
$latex P=(X-z_0)Q,$
so that $latex Q$ has degree $latex n-1$ and has zero set $latex Z$ formed of the zeros of $latex P$ different from $latex z_0$ (since the latter is assumed to be simple). Using the definition, we have
$latex Q'(z_0)=P'(z_0),\quad\quad Q”(z_0)=\frac{1}{2}P”(z_0).$
We now compute the value at $latex z_0$ of the logarithmic derivative of $latex Q$, which is well-defined: we have
$latex \frac{Q’}{Q}=\sum_{\alpha\in Z}\frac{1}{X-\alpha},$
hence
$latex \frac{Q’}{Q}(z_0)=\sum_{\alpha\in Z}\frac{1}{z_0-\alpha},$
which becomes, by the above formulas and the definition of $latex w_0$, the identity
$latex \frac{1}{z_0-w_0}=\frac{1}{n-1}\sum_{\alpha\in Z}\frac{1}{z_0-\alpha},$
or equivalently
$latex \gamma(w_0)=\frac{1}{n-1}\sum_{\alpha\in Z}{\gamma(\alpha)},$
where $latex \gamma(z)=1/(z_0-z)$ is a Möbius transformation.

Recalling that $latex |Z|=n-1$, this means that $latex \gamma(w_0)$ is the average of the $latex \gamma(\alpha)$. It is then elementary that for line $latex L$, either $latex \gamma(Z)$ is contained in $latex L$, or $latex \gamma(Z)$ intersects both components of the complement of $latex L$. Now apply $latex \gamma^{-1}$ to this assertion: one gets that either $latex Z$ is contained in $latex \gamma^{-1}(L)$, or $latex Z$ intersects both components of the complement of $latex \gamma^{-1}(L)$. We are now done, after observing that the lines passing through $latex \gamma(w_0)$ are precisely the images under $latex \gamma$ of the circles and lines passing through $latex w_0$ and through $latex z_0$ (because $latex \gamma(z_0)=\infty$, and each line passes through $latex \infty$ in the projective line.)